Now that you have learned the importance of arrays and learned how to build them for one digit and two digit numbers, let’s take a look at how arrays can also help support students’ understanding of division.

When we build a multiplication array, we are showing the factors through the length and width.  We are showing the product with the total number of squares in our array.  To solve a division problem, we work in reverse.  We start with our dividend or the total number.  Then we can organize those pieces into rows based on our divisor.  Then, once we have passed out all the pieces, we will know how many columns we have and we will know our quotient.

Division Array Model

Take a look at how that works, step by step.  We will consider the problem 182 ÷ 13. We'll start by getting out 182 pieces.

Since our divisor is 13 we want to start arranging our pieces into 13 rows. We don't yet know how many columns we will need. Once we have 13 rows, we can use the extra pieces to start making our columns. We will keep adding columns until we have used up all the pieces and they are in the shape of a square or a rectangle.

breaking our ten into units

the completed array is in the shape of a rectangle

Division Arrays With Remainders

What about remainders? We may reach a point in building our array where there are some pieces left over. In this case there are not enough to create a new column. These pieces are our remainder.

In the example of 59÷4 we have 59 squares that we need to arrange into four rows. After creating our four rows we have 19 squares left. We can put 16 of them into four new columns but we still will have 3 left over. This is not enough to create a whole new column so this is our remainder.

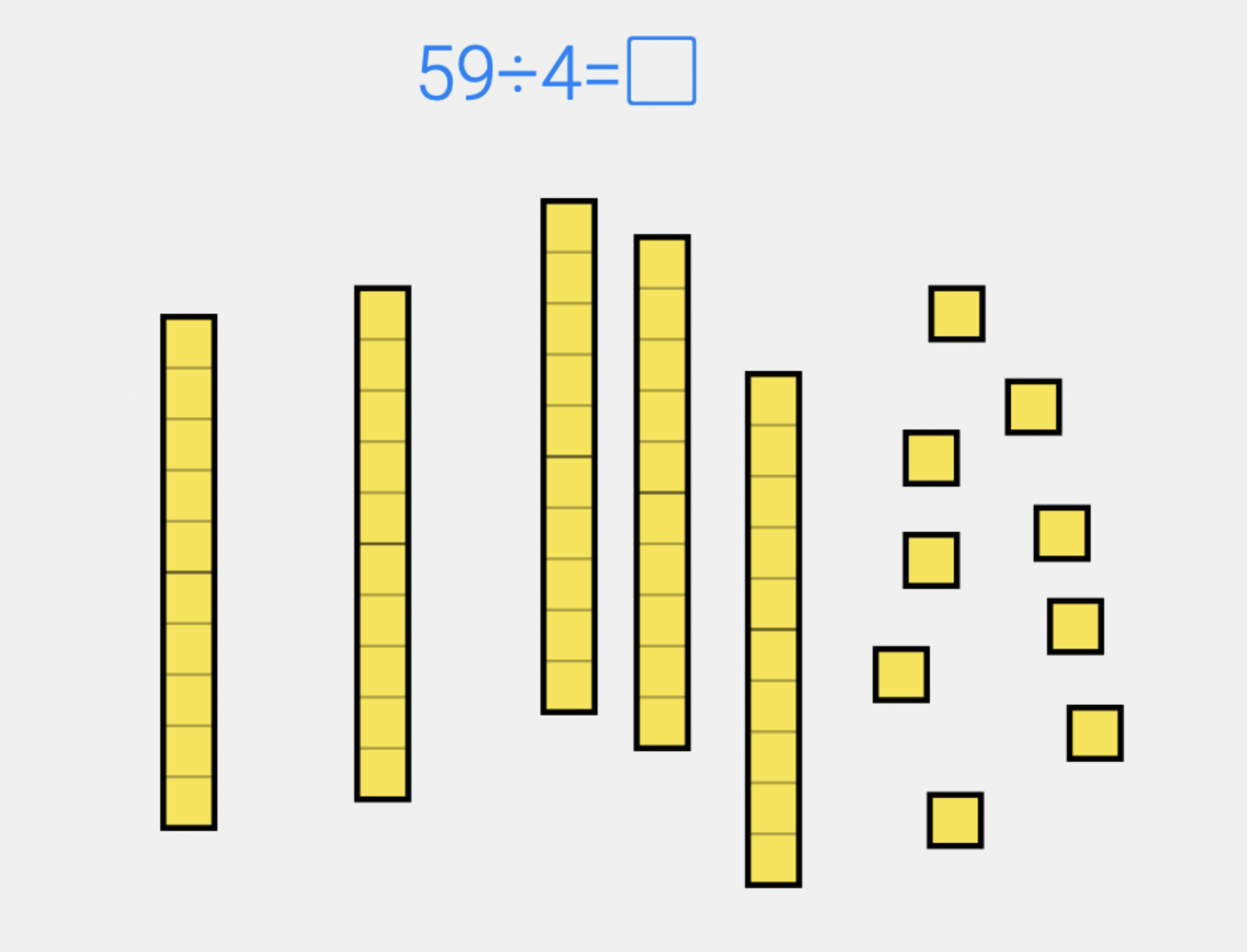
Be careful as the biggest mistake students make when building their arrays is that they keep adding pieces to make the array into a square or a rectangle.  For example, in the problem above a student might be tempted to just get one more square so that they can create another column. If they do this, however, they will be solving 60÷4 rather than 59÷4. A quick reminder to check their dividend and make sure they do not have more than this usually clears up this error. 

Building Conceptual Understanding of Division

I once spent a long time talking with a fifth grade class about division. I asked them what “division” meant.  A few could do long division.  Many could write a division problem.  At the same time, few had any idea what it meant to divide.  By modeling division with arrays, students build an understanding that they are sorting a number into equal groups.  

When students build arrays to represent division problems, they develop an understanding of the concept of division.  It makes sense to them and becomes much more than a memorized process (think long division!!!) that few can remember and many come to despise.  With an array, students can keep track of the pieces of the problem and the work they are doing makes sense. 

Build some of your own division arrays using the Math Learning Center Number Pieces App.